Numeracy Experience Session 3: Exploring Numbers: Concepts and Sense (25/09/13)

Arrrgghhh! Quiz Day! I was so nervous about the quiz before I even stepped into the class. It’s been quite some time since I attempted a quiz or test, and of course today was not a day that I was looking forward to. However, when I saw Dr Yeap’s written schedule on the board, I was quite relieved when I saw the quiz was slotted just before break time. Okay, at least it’ll give me time to gain composure and relieve the butterflies in my stomach for now.

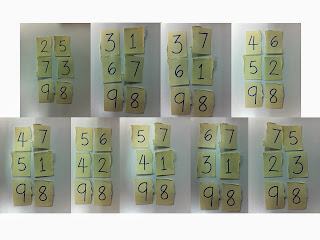
I’ve always enjoyed manipulating with numbers, especially the first problem for today: "With numbers from 0-9, use any 2-digit number and add with another 2-digit number, to still get a 2-digit number". As I was doing this activity, I explored with many digits to ensure that they are not only, not repeated, but also to ensure that the outcome is still a 2-digit number. Some of the combination of digits that I attempted was …

  42                    43                   28       
+36                 +25                 +65
  78                    68                   93

What makes it interesting as I explored the combinations, I discovered 9 ways (there could be more) of combining digits and eventually resulted into an outcome of 98. Amazing, right?!

When Dr Yeap shared his other findings on how to solve the above problem, he pointed out something interesting which I have never took note of until tonight. He said, “When teaching Mathematics, do not leave out the nouns. For example, when teaching 25 + 12, instead of saying ‘what is 5+2 and 2+1’ to the children, teacher should say ‘what are 5 ones and 2 ones; what are 2 tens and 1 ten?”.

I thought what he said made sense to me. This will be one way to get out children to be familiarized with the terms ‘ones’ and ‘tens’ even if they are not doing on that topic. In fact, it will help the children to quickly think of the outcome instead of having to do the traditional method of the ‘carry 1’ over to the tens when the number of ones is more than 10.

ALMOST BREAK TIME! Of course, it also meant that it’s quiz time! Trust me, I was so nervous while waiting for the paper to reach me. However, the moment I turned the paper over, I saw a familiar question: a repeat of what I went through in Session 1 i.e. the letter that falls in the 99th position. This time round, it’s changed to ‘the letter that falls in the 2013th position’. Challenging but of course, there’s surely a smarter way of doing it. How did I do it? Do I need to explain? Why not you try it out and see the outcome?

Quiz over and I thought the nerve-wrecking emotion ended there. My, I was wrong! Dr Yeap actually gave us another problem for us to solve and boy, we spent almost one hour and a half thinking and deriving different ways of splitting a rectangle shape (which we literally assumed for it to be a chocolate) equally amongst 4 persons. Here were some ways that I managed to come up with:

 

 

Conclusion: THROBBING HEADACHE

 

 

 

 

What I discovered from Dr Yeap in today’s session:

Enrichment is not about practice. It is about challenging the child further. It is also not about doing the activity/lesson at another time but it’s about adding the challenge within the same activity/lesson.

Sounds tricky to you? Digest it. I did.

 

Signing off till another note,

Miss Khadijah Senan